Let me take back what I said earlier about finding numerical puzzles easier. I have a great fondness for the number puzzles, my first completed Listener was "Telling Fibs" by Arcturus (a peek at the standings for 2005 show that it was solved by twice as many people as any other puzzle that year, so maybe I shouldn't be boasting).
This time we've got three grids, Fibonacci numbers, primes and cubes. And there must be some logical starting point. Eventually I found it... and it turned out to be my stopping point as well.
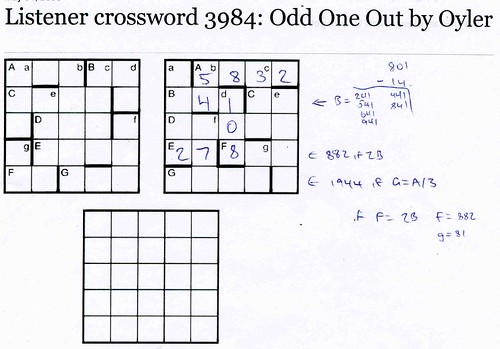
I numbered the grids with the bars 1 and 2, and the empty grid 3...
2C cannot be E+E'+e
2C cannot be Prime x F - so 2C is prime
1D cannot be ExG
2F cannot be A+e'
2B cannot be AB
1c cannot be d'-B (since B is larger than d')
1d cannot be 5G
2d cannot be 5G - so 3d is 5G
1d cannot be A/b
Since 2d is A/b, 2A cannot be prime.
This leaves 2A to be a 4-digit Fibonacci number divisible by either a 2-digit Fibonacci number (none work) or divisible by a 2-digit even number (none work)
Or 2A is a cube divisible by a 2-digit Fibonacci number (no), or by an even 2-digit number that starts with the same number. That gives me the trivial case of 8000 (can't be 8000 since that would make 2c start with 0), or 5832 which is divisible by 54 to get 108. Woohoo, I'm on a roll and I can fill in 2A, 2b, 2d, and 2E
And that got me to a complete and utter grinding halt.
Oyler beats me again - I got really close on last year's "Dedication".
The gap is closing... current tally: Listener 10, George 12. Current streak: Listener 2

2 comments:
Abject failure for me too. I was happy to be taking this one away for our week's holiday (no Chambers needed!) and made insufficient progress to be able to enter any answer. Sadly, I am a graduate mathematician!
This is one week that I do feel dissatisfied after reading the solution. I don't think the preamble was clear, it said "No two bars across all three grids are in the same grid position." To me that doesn't mean "No two grids have bars in the same position". If that was clearer, then being able to draw the gridlines on C straight away makes it a much much easier problem, and I probably would have finished this.
Post a Comment